Let us now have a brief look at the details of the model. The building blocks for describing firm behaviour and demand have remained unchanged. We still employ the equations and parameter values described previously.

What has changed is that we have now taken this model to the next stage: instead of ‘only’ describing firm behaviour, it is now an equilibrium model that describes firm behaviour, consumer behaviour, and the linkage of these two implied by market clearing. The model not only calculates how much electricity each firm produces and how much electricity consumers demand for given prices and policies but it matches aggregate supply and demand by adjusting the market price for electricity.

How does this work? You have to add a balance between supply and demand, like in the very first model exercise.

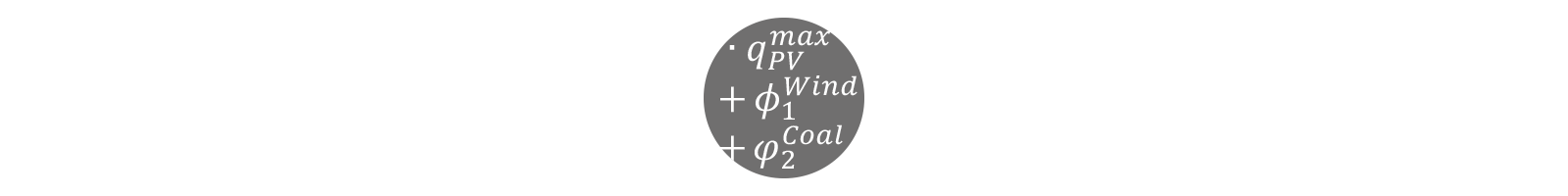
First, let’s define the production of each firm, $$i$$, (ie, the $$q_i$$) for a given price and sum them to obtain the aggregate production of all the firms at that price, $$Q^{supply}$$:

---

$$Q^{supply} = \sum_{\begin{gather}i=firm_{PV},firm_{Wind},firm_{Coal} \ firm_{Gas}, firm_{Nuclear}\end{gather}}q_i$$

---

Second, demand is still given by

---

$$d= \overline{Q}-\eta \cdot p$$

---

Now, you can add a balancing equation that models the fact that an electricity market must clear (electricity demand has to equal supply):

---

(1) $$d=Q^{supply}$$.

---

This additional equation determines the electricity price, which, so far, has been an exogenous variable. Now, it becomes endogenous.

Note that equation (1) cannot be easily solved analytically, as the equations for the different firms are too complex. Thus we use a numerical algorithm to solve this equation.

This is the first equilibrium model that we have used in this course. These models are important as they describe how a society consisting of different actors (firms, in our case) work if the connections between actors are taken into account. From now on, we will always use this type of model, as connected actors are what we usually encounter in modelling future energy systems.